ezyang’s blog

It's been a year since I got my hood and gown and joined Facebook (where I've been working on PyTorch), but while I've been at Facebook Backpack hasn't been sleeping; in fact, there's been plenty of activity, more than I could have ever hoped for. I wanted to summarize some of the goings on in this blog post.

A run-time debugger allows you to see concrete values in a program, make changes to them, and continue running your program. A compile-time debugger allows you to see symbolic values in a program, reason about them, and write the rest of your program, e.g. filling in missing tensor size checks, for example.

Here's an example of a compiler-time debugger in action.

Let's suppose you are writing a simple program to read a pair of tensors from two files and do a matrix multiply on them. "Easy," you think, while writing the following program:

main() {
  x = load("tensor1.t")
  y = load("tensor2.t")
  return matmul(x, y)
}

However, there is a twist: this matrix multiply is an unchecked matrix multiply. If you pass it tensors which cannot be validly multiplied together, this is undefined behavior. Your compiler has cottoned up to this fact and is refusing to compile your program. You fire up your compile-time debugger, and it drops you to the point of your program right before the error:

# Ahoy there Edward!  I stopped your program, because I could not
# prove that execution of this line was definitely safe:

   main() {
     x = load("tensor1.t")
     y = load("tensor2.t")
->   return matmul(x, y)
   }

# Here's what's in scope:

  _x_size : List(Nat)  # erases to x.size()
  _y_size : List(Nat)  # erases to y.size()
  x : Tensor(_x_size)
  y : Tensor(_y_size)

# I don't know anything else about these values

Let's take a careful look at the variables in scope. Our compile-time debugger tells us the type of a variable x by writing x : t, meaning that x has type t. We have all sorts of ordinary types like natural numbers (Nat) and lists of natural numbers (List(Nat)). More interestingly, a tensor is parametrized by a list of natural numbers, which specify their sizes at each dimension. (For simplicity, the underlying field of the tensor is assumed to be fixed.)

Our debugger has a command line, so we can ask it questions about the types of things in our program (:t is for type):

> :t 1
# Here's the type of 1, which you requested:
Nat

> :t [1, 2, 0]
# Here's the type of [1, 2, 0], which you requested:
List(Nat)

> :t matmul
# Here's the type of matmul, which you requested:
forall (a, b, c : Nat). (Tensor([a, b]), Tensor([b, c])) -> Tensor([a, c])

The type of matrix multiplication should make sense. We say a matrix multiply takes two 2-D tensors of sizes AxB and BxC, and produces a tensor of size AxC. An equivalent way of phrasing, as was done in the type above, is to say, “for any natural numbers A, B and C, matrix multiply will take a tensor of size AxB and a tensor of BxC, and give you a tensor of size AxC”.

It is also instructive to see what the type of load is:

> :t load
# Here's the type of load, which you requested:
String ~> exists (size : List(Nat)). Tensor(size)

We do not know what the dimensions of a tensor loaded from a file are; all we can say is that there exists some size (list of natural numbers), which describes the tensor in question. Our compile-time debugger has helpfully given us names for the sizes of our tensors in scope, _x_size and _y_size, and has also told us how to compute them at runtime (x.size() and y.size()).

Enough of this. Let's remind ourselves why our program has failed to typecheck:

> matmul(x, y)

# I'm sorry!  I was trying to find values of a, b and c which
# would make the following equations true:
#
#     [a, b] = _x_size
#     [b, c] = _y_size
#
# But I don't know anything about _x_size or _y_size (they are skolem
# variables), so I couldn't do it.  Cowardly bailing out!

The compiler is absolutely right. We don't know anything about the size of x or y; they could be 2D, or they could be 100D, or not have matching dimensions.

As an aside: sometimes, it's OK not to know anything about the sizes. Consider the case of adding a tensor to itself:

> add
# Here's the type of add, which you requested!
add : forall (size : List(Nat)). Tensor(size) -> Tensor(size) -> Tensor(size)

> add(x, x)
Tensor(_x_size)

# This type-checked OK!  I set size = _x_size and all of the arguments
# checked out.  You're good to go.

We don't know anything about _x_size, but add doesn't care; it'll take any List(Nat), and _x_size is certainly one of those.

Back to business. We are going to insert dynamic checks will will refine our knowledge of x and y, until it is obvious that matrix multiply will succeed.

What is a dynamic check? Operationally, a dynamic check tests whether or not some condition is true, and aborts if it is not. If we successfully run the dynamic check, we now have new information about the symbolic types in our scope. So for example, after adding a runtime test that two numbers are equal, we can subsequently assume at compile time that the numbers are equal:

> :t assert_eq_nat!
(x : Nat) -> (y : Nat) ~> x = y

First things first, we'd like to assert that our tensors are 2D tensors:

> assert_eq_nat!(len(_x_size), 2)

# OK!  I added assert_eq_nat!(len(x.size()), 2) to your program, and
# here's what I know now:

  _x_size : List(Nat)
  _y_size : List(Nat)
  x : Tensor(_x_size)
  y : Tensor(_y_size)
  len(_x_size) = 2

# By the way, I can profitably destruct _x_size into its constituent
# parts; would you like to do this? (Y/n)

> Y

# OK, your new context is this:

  _x0, _x1 : Nat
  _y_size : List(Nat)
  x : Tensor([_x0, _x1])
  y : Tensor(_y_size)

# I don't know anything about the new variables _x0 and _x1, but I
# learned enough about _x_size that I was able to eliminate it from
# the context (_x_size = [_x0, _x1])

List length is a very helpful property to test against, since it greatly specifies the shape of the list in question. We can do the same for _y_size:

> assert_eq_nat!(len(_y_size), 2)

# OK!  I added assert_eq_nat!(len(y.size()), 2) to your program, and
# here's what I know now:

  _x0, _x1 : Nat  # erases to x.size(0), x.size(1)
  _y_size : List(Nat)
  x : Tensor([_x0, _x1])
  y : Tensor(_y_size)
  len(_y_size) = 2

# By the way, I can profitably destruct _y_size into its constituent
# parts; would you like to do this? (Y/n)

> Y

# OK, your new context is this:

  _x0, _x1 : Nat  # erases to x.size(0), x.size(1)
  _y0, _y1 : Nat  # erases to y.size(0), y.size(1)
  x : Tensor([_x0, _x1])
  y : Tensor([_y0, _y1])

# I don't know anything about the new variables _y0 and _y1, but I
# learned enough about _y_size  that I was able to eliminate it from
# the context (_y_size = [_y0, _y1])

We're very close now. All we need to do is assert that the inner dimensions are equal:

> assert_eq_nat!(_x1, _y0)

# OK!  I added assert_eq_nat!(x.size(1), y.size(0)) to your program.
# After doing this, I learned _x1 = _y0, so I replaced all occurrences
# of _y0 with _x1. Now the context looks like this.

  _x0, _x1 : Nat  # erases to x.size(0), x.size(1)
  _y1 : Nat  # erases to y1.size(1)
  x : Tensor([_x0, _x1])
  y : Tensor([_x1, _y1])

Victory!

> matmul(x, y)

# This type-checked OK!  I set a = _x0, b = _x1, c = _y1 and all of the
# arguments checked out.  You're good to go.

Extracting the contents of this session back into our code, we now have:

  main() {
    x = load("tensor1.t")
    y = load("tensor2.t")
    assert_eq_nat!(x.size(), 2)
    assert_eq_nat!(y.size(), 2)
    assert_eq_nat!(x.size(1), y.size(0))
    matmul(x, y)
}

---

At this point, I must come clean: the compile time debugger I've described above doesn't actually exist. But it is not all that different from the proof modes of interactive proof assistants the automated theorem proving community works with today. But unlike theorem proving, we have a secret weapon: when the going gets tough, the tough turns into a runtime check. Conventional wisdom says that automated theorem proving requires too idealized a setting to be useful in writing software today. Conventional wisdom is wrong.

Raise your hand if you've ever put one of these commands in your continuous integration scripts:

• apt install somepackage
• pip install -r requirements.txt or pip install somepkg
• conda install blah
• cabal update or cabal install blah
• git clone https://github.com/someguy/somerepo
• wget http://some-website/thingy-latest.tgz

Can you tell what the problem is? These commands are not reproducible: depending on when you run them, they may give different results. More insidiously, most of the time they give you the same result (or, perhaps, a different result that still works for your use case).

I know, we need a reproducible build! The prevailing answer to this problem by tooling authors has been to seize the means of production and replace it with something that is reproducible. If you live the npm/yarn ecosystem, lockfiles ensure all of your dependencies redownload the same way every time (except when it doesn't). If you live in the Stack ecosystem, Stackage distributions ensure that you get the same Hackage package every time you build (except when it doesn't...). If you live in the Nix ecosystem, it means literally replacing the packaging system for everything on your system to achieve reproducibility.

So, it seems:

1. If you can live entirely within the walled garden of the tools you use, things are pretty reproducible, but you're still on your own when it comes to taking updates on your dependencies.
2. As soon as you step outside of the garden, it's entirely up to you to ensure reproducibility. The "easy way" out is usually not reproducible.

What if we change the question? We have entered this discussion under the assumption that reproducibility is our terminal value. But it's not: it's the mechanism by which we can achieve other goals. In the setting of continuous integration, what we really care about is a system that gives us signal about whether or not a given change set is correct or breaks things. A non-reproducible build interferes with this goal only in the sense that's its harder to tell if a change set has broken things if some random dependency has self-updated itself and broken your build. If this happens, you are blocked: you won't get clean signal until you fix the dependency problem. Broken window theory demands you drop everything and fix the build.

Clearly, we don't care if our dependencies are getting silently upgraded as development proceeds; in fact, we might prefer it, because "automatic" is less friction than "manual", at least when it works. What we do care about is the ability to block the upgrade if it is known to break us or revert the upgrade if we find out later that it caused some breakage.

Online/offline continuous integration. We traditionally think of the continuous integration build as a single pipeline which, when run from beginning to end, gives us signal about whether or not our code works or not. But I think it is better to think of a CI pipeline as dividing into two phases:

1. Online environment configuration. In this stage, you download all of the external software that depend on that fiddly third-party world, setting up a complete build environment. Once you are done, you snapshot this environment by some mechanism (e.g., filesystem snapshot or make a Docker image.)
2. Offline actual build and test. In this stage, within the snapshotted environment from step (1), turn off your Internet connection and run the actual build and test.

The key is that you don't have to run step (1) every build (you didn't want to anyway, for performance reasons.) Instead, the series of immutable snapshots of build environments generated by step (1) gives you the ability to revert or peg to a particular version of all of your dependencies, without having to go and make the universe reproducible. You can have a weekly cronjob rebuilding your environment, running the tests, and only deciding to push the activate snapshot forward if everything passes. You don't have to actually turn off the Internet when you run step (2), but it might help keep you honest.

Think offline. In today's connected world, it's easy to build systems with the assumption that you are always connected to the Internet. Doing so, however, leaves your tool at the mercy of the sound and fury of the real world. By applying a simple principle: "what can I do offline; what must I do online?" we reverse-engineer a design for continuous integration that gives you something almost as good as reproducibility, without forcing you to rewrite the universe. Surely that's worth something.

The best and worst thing about semantic import versioning is that it makes BC-breaking changes hard.

In the past few days, Russ Cox has made a splash in a series of white papers describing Go and Versioning. In them, he coins a new term, Semantic Import Versioning, distilling it to the following principle:

If an old package and a new package have the same import path, the new package must be backwards compatible with the old package.

I am very happy Russ has come up with a good name for semantic import versioning, because this concept has been out there for quite a long time, but without a concise name or formulation of its the design. In fact, I would even say that semantic import versioning is inevitable when you take on the premise that you will never break user code. It is so inevitable, that semantic import versioning is already practiced in the wild in a variety of places. Here are a few examples:

• REST APIs often are versioned with explicit version numbers in the request (e.g., in the URI) to let clients specify what version of the API they want. If a client wishes to upgrade to a new version of the API, they must rewrite their API requests to a new URL. REST APIs are forced to semantic import versioning because the traditional mechanism for avoiding breakage, version bounds, are unavailable in this setting.
• Stripe's REST API pins each of their customers to the version of their API at the time they subscribed; even if Stripe makes a BC-breaking change in the future, the API for a given customer never changes. In this case, the semantic import is still there, but it is implicit (associated with a customer account) rather than explicit (in the client code); consequently, Stripe is willing to break BC a lot more frequently than would otherwise be acceptable for a REST API. Stripe's blog post points out a very important aspect of maintaining libraries under semantic import versioning, which is that you need to put in the engineering effort to sustainably manage all of the semantic imports available to users.
• Semantic import versioning is widely practiced in programming languages, in the form of language standards/epochs. In C++, the setting of -std=c++xx specifies a particular semantic version to be "imported". It would be unheard of for a compiler to unilaterally break backwards compatibility of -std=c++11 in a new revision of the compiler; similarly, a user must explicitly migrate to a new language standard to take advantage of any new features. Rust epochs have a similar tenor. The choice between Python 2 and Python 3 is another form of semantic import versioning.
• Semantic imports don't have to just specify a number. Feature flags, such as {-# LANGUAGE #-} pragmas in GHC Haskell, let users opt into BC-breaking changes at their use-sites.
• In the deep learning world, ONNX models declare a semantic import to a particular version of an operator set. Operator semantics can evolve in BC-compatible ways without bumping the version, but to take a BC-breaking change, you must update the import statement.

One insight I draw from these examples is that what we call an "import version" is really a specification for some series of implementations. To someone who has spent a lot of time thinking about module systems, this is really a step in the right direction: program against interfaces, not implementations.

Another thing we can observe from these examples are the real world consequences of semantic import versioning. One particular effect stands out: semantic import versioning is challenging for maintainers, because it pressures them to maintain multiple major release branches simultaneously (after all, who wants to use pkg/v2 only to have it immediately unmaintained when pkg/v3 comes out). In the traditional release branch model, where one creates a release branch for each major version, only the most well-staffed software development teams can afford to maintain multiple, active release branches (backporting patches is a lot of work!) The friction involved with managing multiple implementations means that less well staffed projects will have a strong pressure to never break backwards compatibility.

This may not sound like a such a bad thing to the "don't break my stuff" grumps in the audience, but a lot of bugs and security problems have stemmed from being literally unable to outlaw harmful and dangerous APIs with BC-breaking changes. The danger of moving the calculus further towards preserving backwards compatibility is a further entrenchment of bad "first try" APIs. So while I do not deny that a genius of Russ's framing is to describe semantic versioning as part of the package path, it also sets up a bad expectation for the feasibility of BC-breaking changes, when what we should be doing is improving the state of tooling so that making a BC-breaking change is "no big deal." To me, the most promising way to reduce the friction of a BC-breaking change is to organize your software development so that a single codebase, under a single build, implements multiple specifications (v1, v2 and v3). As we saw from the examples, compilers can manage this (GCC supports multiple C++ versions), but traditional programming languages make it hard for libraries to do the same thing.

I don't now exactly how to solve this problem, but I do have a few ideas:

1. Treat specifications as data. This means you can write code that operates over a specification, and for example, automatically generate the boilerplate necessary to forward from one implementation to another.
2. Don't ask programmers to manually write diffs. I would never ask you to make a source code change by writing a diff by hand, just because this is the best representation for a VCS to store. Instead, you would just make the edit, and expect the system to figure it out. BC-breaking changes to APIs follow the same principle; it is much simpler and easy to understand if you just make the change, rather than write a description of the change
3. Package level modularity. In a traditional package management system, I can't release a single bundle of source code which presents multiple "package interfaces". Even in vgo, even if I have a shared codebase implementing v1 and v2, I still have to make two releases to publish a new version of code. This is backwards; there is no reason a single unit of code cannot provide multiple interfaces, and package tools should make this possible.

These are maybe a bit too radical to expect Go to adopt them, but perhaps the next generation of programming languages will explore this design space further.

• JG: Joseph Gonzalez
• GG: Garth Gibson (CMU)
• DS: Dawn Song (UC Berkeley)
• JL: John Langford (Microsoft NY)j
• YQ: Yangqing Jia (Facebook)
• SB: Sarah Bird
• M: Moderator
• A: Audience

M: This workshop is bringing together ML and systems. Can you put your place on that spectrum? Who is your home community?

YJ: Right in the middle. I'd like to move more towards systems side, but Berkeley Parallel Labs kicked me out. ML is my home base.

JL: ML is where I come from, and where I will be, but I'm interested in systems. My home is NIPS and ICML

DS: My area is AI and security, did computer security in the past, now moving into AI.

GG: Systems.

JG: I started out in ML, working on probabilistic methods. I basically, in middle of PhD, looked at systems. Now I'm moving to being a systems person that does ML.

M: We've seen a proliferation of deep learning / ML frameworks that require a lot of dev effort, money, time to put in. Q, what is the role of academia of doing research in this area. What kind of large scale ML learning can you do.

GG: I liked YJ's answer last time.

YJ: The thing that is astonishing is that academia is the source of so many innovations. With all due respect, we did very good work in Google, but then Alex came out with 2 GPUs and nuked the field. Academia is the amazing place where we find all of the new ideas, and industry scale it out.

JL: Some examples. If you're coming from academia, maybe you don't have research at big company, but it's an advantage as you will spend time about the right algorithm for solving it efficiently. And that's what will win in the long run. Short term, they'll brute force with AutoML. Long run, the learning algorithms are going to be designed where tjhey won't have parameters. A common ML paper is "we eliminate this hyperparameter". When they're more automatic, more efficient, great things will happen. There's an advantage in being resource constrained, as you will solve things in the right way.

Another example is, the study of machine learning tells us that in thefuture we will regard any model that u just learned and deploy as inherently broken adn buggy as data collection is not part of process of training, deploying. It will decay and become irrelevant. The overall paradagim of ML where you're interacting with the world, and learning, that can be studied easy in academia, and that has huge implications about how you're going to design systems,

DS: People often talk about in a startup, the best thing is to not raise a ton of money; if you're resource constrained you're more focused and creative. ML is really broad, there's lots of problems. Right now we learn from lots of data, but lots of talks at NIPS, humans have amazing ability to learn from very few example. These are problems for academia to tackle, given unique resource constraints.

GG: I'll say, it's difficult to concentrate on top accuracy if you don't have enough data, and the data available to students is stuff like DAWNbench which tends to lag. In academia, we build relationships with industry, send students for internships, they get the ability to do big data, while exploring first principles in university. IT's a challenge, but open publishing and open sharing of code world more berable.

JG: The one thing I've struggled with is focusing on human resources. I have grad students; good students, focus on a key problem can make a lot of progress. We struggle with a lot of data. Struggle with RL really is here, we can build simulators to build at this scale. Being able to use simualtion to get data; be creative, find new and interesting problems.

M: Follow-up on process. I think a lot of you have tried to publish ML in your communities. Are they equipped to appreciate work properly; what is a common reason they don't appreciate.

JG: Publishing ML in systems, or vice versa, is hard. It goes both ways. These communities are not equipped to evaluate work in other field. ML in systems, where if you saw here, it was surprising. Or vice versa, wouldn't have done well in systems venue as systems. The failure mode I see, is systems community doesn't appreciate extreme complexity. In ML, I have this very sophisticated thing, and reducing them to their essential components. ML tries to overextend their complexity as an innovation. MOre broadly, each of these communities has their own biases how they look at research. One thing I've noticed, it's gotten better. Systems is better at evaluating, and at this workshop, people are pushing research in an advanced way.

GG: I'm old, so I've seen creation of conference before. So, you start off with an overlap of areas. In my prior life, it was the notion of storage as a research area, rather than app of devices. You start off, send submission in. The PC has two people that know anything about it, and they aren't assigned, and the reviews are sloppy, and you get one conference that do a little better, but other conferences don't read it. I faced this with fault tolerance, database, OS communities, they don't read each other's stuff. You get enough mass, get a conference that focuses in the middle; reviewing and PC that have seen most of the good work in the area. That's hard, but we're on the edge of doing it in SysML. We're doing the right thing to do competitive, on top of state of the art.

M: Is that the only solution, or can we mix up PCs?

GG: I've seen a lot of experiments to try it. You can end up with permanently fractured communities.

JL: Joey and Dawn are an area chair at ICML. I have found the ML community to be friendly to system type things. There's an area chair systems. Hopefully papers get assigned appropriately.

M: We're not good about that at systems.

DS: About ML and security, we have this problem. In security, we also have very small percentage of ML, and the committee, if you submit ML, it's very hard to find people who can review the paper, and as a consequence, the review quality varies highly. Similar in terms of security in ML, similar problems. It's interesting to think about why this happens and how to solve the problem. In general, sometimes the most interesting work is the interdisciplinary areas. ML and systems, security, and examples I see, including machine learning in systems... so, one thing I actually can understand is, within each community, even though the review quality varies, I can see from committee's perspective, really what they want is papers that are more meaningful to community, help people get exposed to this new area, fostering new exploration. That's part of natural progression. As time goes on, there's more cross pollonization.

JG: We are launching a SysML conference. I had a little bit of reservations: ML is getting better at systems, but now I have to decide where I'm going to send a paper. A lot of papers we see in ML is going to have systems.

GG: When you have a new conference area, not all work is sent there. Overlapping, you have a favorite conference, your heros, and you'll send your most exciting work to that root conference. No problem.

YJ: SysML is great, and this is how it comes out. New fields, it warrants new conferences.

M: Do you think ML expert needs to also be a systems expert? Does such a person who lies at that intersection have a different way of looking? Or you come up with a nice algorithm, and you

JL: It's not OK to have a wall.

There's many way learning algorithms can be changed. The problem with having a wall, if you don't understand, throw engineer. But if you can bridge to understand, they're not artifacts, you can break open and modify. That can let you achieve much better solutions.

GG: AGreed, but what happens initially is you reach over to other side, you put it into system, and it's my innovation that redundancy makes fault tolerance, even though it's fairly pedestrian from the other side. If it is a substantial improvement, it is worth doing. We all grow up.

JG: We need a wall, but we're going to constantly tear it down. Matlab in grad school, we made jokes about it, and MKL community would make it fast. Then they said we are going to build ML for distributed computing algorithms, and ML would write class algorithms for system. That waned in the dev of pytorch, TF, etc., which leveled up abstraction. The stack is building up again; systems community to make more efficient. Well, fp could change, and that could affect algorithm. So we're tearing it down again. But systems is about designing the wall.

YJ: It's more like a bar stool. It's a barrier, but we don't have to be both to do anything, but you need it to make it efficient. A story: a training system we looked at, SGD. That person found a very nicely rounded number: 100. But people frown, you should round to 128. Understanding and improving the common core for CS and engineering, that helps a lot for people to have good sense for how to design ML algorithms.

M: There's a lot of talk about democratizing AI, and all of you have helped that process. What is a truly democratic AI landscape look like, and how far are we from that world.

YJ: I plead guilty in participating in framework wars. When reading CS history, one thing that's pretty natural, when field is strating, there's all sorts of standards, protocols. FTP, Gopher, and now in the end HTTP took over, and everything runs on HTTP. Right now, there's all kinds of different abstractions; boiling it down, everyone is doing computation graph, optimization. I look forward to when we have one really nice graph representation, protocol for optimizing graphs. It's not a rosy dream, because in compilers we have that solution, LLVM. I don't know if we'll reach that state but I think one day we'll get there.

JL: You have AI/ML democratized when anyone can use it. What does that mean, a programmer has a library, or language constructs, which that they use routinely and easily; no issues of data getting mismatched or confused or biased. All the bugs people worry about in data science; those are removed from the system because the system is designed right and easy to use. The level beyond that is when somebody is using a system, that system is learning to adapt to you. There's huge room for improvement in how people interact. I don't know how often there's a rewrite rule driving me crazy; why can't it rewrite the way I want. People can signal info to a learning algorithm, and when those can be used effectively tpo assist people, you have democratized AI.

DS: I have a very different view of democratizing AI. I think it's interesting to think about what democratization here really means. For systems people, it's about making it easier for people to do learning, to use these libraries, platforms. But that's really just providing them with tools. For me, I give talks on demccratizing AI, we are looking at it from a completely different perspective. Code: even, whoever controls AI will control the world. So who controls AI? Even if you give everyone the tools, push a button, but they don't have the data to do the training. So who controls the AI today, and tomorrow? It's Facebook, Microsoft, Google... so for me, democratization means something totally different. Today, they collect data, train models, and they control who has action to model, and users can get recommendations, but not direct access to models. We have a project to actually democratize AI, where users can control their data. Combining blockchain and AI, where users can donate their data to a smart contract, where the smart contract will specify the terms; e.g., if you train a model, the user can use the model, and if the model produces profits, the user can get part of the profits. The smart contract can specify various incentive terms; e.g., if the data is vbetter than others, they can get more profits, and other mechanisms. A developer will supply the ML training algorithm, and get benefits when it is trained well. We are decentralizing th epower of AI; users will be able to get direct access to models and use them. In this case, I hope for an alternate future, where big companies can continue with business, but users by pooling their data in a decentralized fashion, will see actual true democratization of AI; they will access the power of AI. Not just use tools.

(applause)

GG: I think that a lot of what's meant in democratizing AI is how can you move from a small number of people innovating, to a large number. Tool development and standards. We're close to being there. There was an example in the past, was VSLI paint boxes. Up until a certain point, only an EE could really develop hardware at all. They took a lot of effort and time to make sure it could make it through very part without very much crosstalk. a group came together and thought, well, there are some design rules. This lets you build hardware pretty easily. I could paint green/red boxes, hardware months later, worked. It never worked as fast as that EE guy, so there would always be a place for it, but it would let us build a RISC computer, and ship it. We were in the game, we could innvoate, and do it. The tools we're trying to build right now can build on statistical.

JG: When I started PhD, we did integrals and derivatives by hand. Automatic differentiation was a huge step forward. I blame that for the explosion of papers. A first year can build something far more complex than what I could do. That's moving AI forward, on algorithms side.

The data side is interesting, and that is one where I think about in systems. There's a lot of opportunities to think about how security interacts, leveraging hardware to protect it, markets to sell/buy data from sources, and protect the data across a lot of places. I would argue we're making a substantial amount of progress in how we think about algorithms.

M: When I think about democratizing pervasive AI, recent questions that have been consuming our minds, interpretability, fairness, etc. Can you share... any experience where things like interpretability came up and became a problem, issue, do we have to worry about a lot more in ML, or systems-ML.

JG: My grad students come to me and say the models stop working. I don't know how to fix that; the process is very experimental. Tracking experiments is a big part of the process. We cared a lot about interpretable models, and that meant something very particular. Now it's explainable; we don't need to know what it did exactly, but there needs tob e some connection to what we did. Interpretable, explain computation, it could be related or unrelated to the decision. That's two answers about explainability, and how we debug these systems.

GG: SOSP just happened, and they have ten years of... good copies of everything they submitted. At the end of the conference, Peter Chen took all the PDF files, and did a naive bayes classifier, and saw how well he would predict that it would be accepted. And half the things it predicted to be accepted, would be accepted.

So what did they do? They made ad etector for popular authors. And so what you did is those who had succeeded, they will follow behind. I recognize this problem. You might think that you found a good way, but it's actually Nicolai Zeldovich's paper.

DS: There's a big debate. Some think it's really important, and sometimes, as long as the model works, it's fine. Our brain, we can't really explain how we arrive at certain decisions, but it works fine. And it depends on application. Some applications have stronger requirements for explainability; e.g., law and healthcare, whereas in others it's less required. Also as a whole community, there's a lot we don't understand. We can dtalk about causality, transparenty, all related. As a whole community, we don't really understand what explainability means. Not a good definition. All these concepts are related, we're trying to figure out what's the real core. That's a really good open question.

JL: There's two different interpretations. Can you explain to a person? And that's limited; there's no explainable vision models. The other definition is debuggability. If you want to create complex systems, they need to be debuggable. This is nontrivial with a distributed system, it's nomntriival with ML. If you want to create nontrivial ML systems, yo uhave to figure out why they're not behaving the way you want it to.

DS: Do we debug our brains?

JL: Evolution has done this the hard way for a very long way... a lot of people have bugs in their brains. I know I have bugs. I get an ocular migraine sometimes... very annoying. No, we don't debug our brains, and it's a problem.

YJ: I'm suire there's bugs in my brains; I chased chickens in my grandma's house; the chicken has one spot in its back that if you press it, it just ducks and sits there. It shuts off because of fear. WE humans don't do that. But these bugs, are in our brain as well. Chasing for interpretability helps understand how things work. The old days, deep dream; this line of work started with figuring out what the gradients do, and we propagated back, and we found that direct gradient doesn't work; then we added L1 priors, and then we got pictures. This curiosity has lead to the fact that convnets with random weights are codifying the local correlation; we are hardcoding the structured info in CNNs which we didn't know before. So maybe we will not achieve full interpretability, but some amount of interpretability and creativity will help.

(audience questions)

A: I'd really like to hear what Jeff said about ML for systems. As systems, I'm interested in it, but people have said, you can get far with heuristics.

JL: I think it's exciting.

GG: The index databases, when I read it for reviewing, I went, "Wow! Is that possible?" I think things like that will change the way we do systems. The novelty of the application opens a lot of people's minds. Right now we think of the machine learning tools as being expensive things that repeat what humans do easily that computers don't do well. But that's not what DB index is. We can execute it, but we're not better. But to get it half the size and twice the speed, throwing in another way of thinking about compression through a predictor is a fabulous insight.

JG: I tried to publish in this area for a while. For a while, systems didn't like the idea of complex algorithms in the middle of their system. Now, these days, Systems is like, "ML is cool." But where it's easier to have success, you prediction improves the system, but a bad prediction doesn't break the system. So scheduling, that's good. Where models can boost performance but not hurt. The work in ML to solve systems is successful.

DS: ML for systems is super exciting. I'm personally very excited about this domain, esp. for people who have done systems work, and are interested in AI. ML for systems is an amazing domain of ML. I wouldn't be surprised, I would hope to see, in five years, our systems are more ML driven. A lot of systems have a lot of knobs to tune, trial and error setting, where exactly ML can help. On these amazing techniques, RL, bandits, instead of using bandits to serve ads, we can try to autotune systems. Just like we are seeing AI transforming a lot of application domains, and a lot more intelligent system, old systems, the one we built, should be more intelligent. It's a prediction: It hink we are going to see a lot of work in this domain. I think it will transform systems.

M: I work in this quite a bit. We have some successes with bandits in some settings, but there are settings that are really tough: stateful, choices, decisions influence the future, it makes it hard to apply RL, or the RL techniques take a lot of data. There are challenges, but there are successes. There are a lot of papers that apply RL in caching, resource allocation. The real question is why it's not used in production? I don't know if we have an answer to that, papers do it, it seems to be really good, but it's not that mainstream, esp. having RL all over the place. Why isn't it pervasive. That I don't see.

A: Isn't it because it's not verifiable. You want some kind of verification analysis.

GG: It's called a regression sweep. If you deploy on a lot of systems. There's a lot of money, it has to work. If it falls over, that's a lawsuit. I hired a VP of software. OK, now that I'm in charge, things are going to slow down. Every LoC is bugs, if I want low bug, I stop programmers from writing code, by making the bar very high. This is the thing JOy was talking about; they need a really compelling reason with no downsides, and then they have to pass tests before the pass. So anything stochastic has a high bar.

SB: Another thing that is happening, there aren't that many people who have understanding in both areas. It's really hard to do ML in systems without deep expertise in systems. You really need to understand to explain it.

GG: It wasn't that long since we didn't have hosted services.

M: Guardrails, you constrain the ML system to not suggest something bad. We have a scenario in MS, machines are unresponsive. How long to wait? You can do it in ML. The choices are reasonable, they're never more than the max you'd want to wait.

A: On democratization. There's been a lot of talk about optimizing the models so they can bear the cost. Another is decentralizing data... but there's two very big constraints for systems and models. They cost a lot of money, and there's big variance. Because of cost, if some guy gets into programming, and does research, he won't have resources to do it. So they won't go into engineering; they'll intern at Amazon instead. So if there is some community going into lowering the barrier, demoratizing, what solution is there to get people much more easily? Because there's huge economic costs. People are trying to make huge amounts of money, startups, but there's no... systems have faults with decentralization... there's just a big problem colliding and ML.

JG: We teach data, I teach data science at Berkeley. The summary is, what about the costs of getting into DL? There's cost to train models, GPUs, data, how do I get a freshman in college who is excited about this, chromebook, they can do research and explore opportunities. At Berkeley we have exactly this problem. I teach 200 students, a lot of them are freshmen, chromebook ipad as primary computer. We've built tools using Azure... we run a cloud in Azure, and on these devices they can experiment with models. They get to use pretrained models and appreciate how to ... Someone built a Russian Twitterbot detector, and saw value and opportunity in those. And then they got involved in research projects where they had more funds and tools.

JL: The right interfaces make a huge difference, because they prevent you from having bugs that prevent you from doing things. Also, DL, is all the rage, but framing the problem is more important than the representation you do. If you have the right problem, and a dumb representation, you'll still do something interesting. otherwise, it's just not going to work very well at all.

YJ: As industry, don't be afraid of industry and try it out. Back at Berkeley, when Berkeley AI was using GPUs, the requirement was that you have one project per GPU. We students, framed ten different projects, and we just asked for ten GPUs. NVIDIA came to us and asked, what are you donig. We'll just give you 40 GPUs and do research on that. Nowadays, FAIR has residency, and Google AI has residency, all of these things are creating very nice collaborations between industry and academia, and I want to encourage people to try it out. Industry has funds, academia has talent, marrying those together is an everlasting theme.

A: Going back to where do we go forward in terms of conferences, the future of this workshop; has any decision been made, where we go?

SB: This is work in progress. We're interested in feedback and what you think. We've had this workshop evolving for 10 yrs, with NIPS and iCML. Then we did one with SOSP, excciting. We are now doing a separate conference at Stanford in February. We think there's really an important role to play with workshops colocated with NIPS and ICML. We're still planning to conitnue this series of workshops. There's also a growing amount of systems work in ICML and NIPS, natural expansion to accept that work. The field is growing, and we're going to try several venues, and form a community. If people have ideas.

JG: More people should get involved.

M: We plan to continue this; audience is great, participation is great.

It's a panel, so I have to ask you to predict the future. Tell me something you're really excited... 50-100yrs from now. If you're alive then, I will find you and see if your prediction panned out. Or say what you hope will happen...

YJ: Today we write in Python. Hopefully, we'll write every ML model in one line. Classifier, get a cat.

JL: Right now, people are in a phase where they're getting more and more knobs in learning. ML is all about having less knobs. I believe the ML vision of less knobs. I also believe in democratizing AI. You are constantly turning ... around you, and devs can incorporate learning algorithms into systems. It will be part of tech. It's part of hype cycle. NIPS went through a phase transition. At some point it's gotta go down. When it becomes routine, we're democratizing things.

DS: It's hard to give predictions... I guess, right now, we see ML as an example, we see the waves. Not so long ago, there was the wave of NNs, graphical models, now we're back to NNs. I think... I hope that we... there's a plateauing. Even this year, I have been talking to a lot of great ML researchers, even though one can say there has been more papers written this year, when you hear what people talk about in terms of milestones, many people mentioned milestones from past years. AlexNet, ResNet, ... I do hope that we will see new innovation beyond deep learning. I do teach a DL class, but I hope that we see something beyond DL that can bring us... we need something more, to bring us to the next level.

GG: I'm tempted to point out DL is five years ago, and dotcom era was not more than five years... I think, I'm looking forward to a change in the way CS, science in general, does business, having learned from statistical AI. My favorite one is overfitting. I poorly understood overfitting, in vague stories, until ML hammered what this said. I look forward to the time when students tell me, they stopped writing code, because they were adding parameters... and they added a decent random, iid process for testing code. We're no where near there, but I think it's coming.

JG: I'm looking forward to the return of graphical models... actually not. When we're democratizing AI, but what ultimately happens, we're democratizing technology. I can walk up to Alexa and teach it. Or I can teach my Tesla how to park more appropriately. Tech that can adapt to us because it can learn; when I can explain to a computer what I want. (Star Trek but without a transporter.)

The below is a transcript of a talk by Daniel Lo on BrainWave, at the ML Systems Workshop at NIPS'17.

---

Deploy and serve accelerated DNNs at cloud scale. As we've seen, DNNs have enabled amazing applications. Architectures achieve SoTA on computer vision, language translation and speech recognition. But this is challenging to serve in large-scale interactive because there are latency, cost and power constraints. Also, DNNs are growing larger in size and complexity.

We've seen a Cambrian explosion in startups to solve this problem. Research groups have produced DNN processing units, DPUs, custom hardware solutions to prove high throughput efficient serving of DNNs. We categorize them into two categories: fast DPUs, where the algorithms and applications have to be fixed in at design time, because they're fabbing an ASIC, or a soft DPU, FPGA. But for soft DPUs, we haven't seen them deployed at scale.

To address this, we've been working on Project BrainWave. Solution to deploy large scale DNNs with FPGA-acceleration. We've designed it to be fast, flexible and friendly. High throughput, low latency acceleration using FPGAs. Flexibility with adaptive numerical precision, update to latest AI algorithms with reconfigurable FPGAs. And it's user friendly, because we have a full stack solution, compile CNTK/Caffe/TF and compile them down. This is deployed on our configurable cloud, an outer layer of CPUs, a data center that puts everything together, and a layer of reconfigurable FPGAs.

We've been deployed DNN models. LSTM model that takes tens to hundreds of milliseconds CPU. What we see is the 99th percentile for latency; even at 99 we are able to achieve sub-millisecond latencies. When you get to these levels of acceleration, it's negligible in the E2E pipeline.

Next I'll dive into details. It's a full stack solution. starting with a compiler and runtime that takes model sin high level frameworks and compiles them down to our architecture. A flexible ISA for serving DNNs. We have a throughput, low latency serving. We do this all with persistency at scale, to keep models pinned in FPGA memories. Deployed on our wide deployment of Intel FPGAs using hardware microservices.

To begin with, let's talk about hardware microservices. This is something we presented at Micro. The architecture of reconfigurable cloud is FPGAs sit between CPU and network. CPU can use FPGA locally for acceleration, but because FPGAs are connected over network, they can distribute between them. We have a proprietary network protocol for low latency compute.

We'vec disaggregated FPGA compute plane from CPU. So we can aggregate FPGAs together to form larger accelerators, and you don't have to match the rate of FPGAs to CPUs. You can serve a large number of CPUs with a small cluster of FPGAs, or vice versa.

Next I'll talk about the compiler and runtime. Goal is to make it very easy for ML specialists to do this. The typical ML specialist doesn't know how to program this. Models developed in high level frameworks, compile them down to our architecture. If you compile them down first into an intermediate graph based representation. We split them into portions split on FPGAs, and portions on CPU. When we execute, we also have runtime that handles orchestration and scheduling that handles it between parts.

There are two main categories of DNNs we have to optimize for. DNNs that have very high compute to data ratio, convnets, these are well studied. I'm going to focus on the other class of DNNs, those with less compute to data ratio, e.g. dense layers and RNNs.

The conventional approach to accelerating DNNs on FPGAs, you keep all model parameters in DRAM. When a request comes in, you're going to stream the model parameters of DRAM, and return a request. The issue with this is when you have DNN layers that are memory bandwidth bound, you're limited in how fast you can run this by memory bandwidth; you're not getting full compute capabilities of FPGA. Typically the way to solve this is with batching; you send a number of requests and use the model parameters for all requests. WHile you may achieve good throughput, latency will increase. For realtime services, this violates your SLA. What we want to do is provide high performance at low or no batching.

The way we do this is with persisted Dnets. FPGAs have lots of memory on chip: 10MB memory. Since they're on chip, it's high bandwidth. So we're going to keep the model parameters on the chip, so that when we get one request in, we distribute it across the entire FPGA chip.

The obvious question is, what happens if your model doesn't fit on chip? We take advantage of the hardware microcenter. We'll distribute a single model over multiple FPGAs in the datacenter.

Let's look at the architecture and microarchitecture of the processing unit we developed. The BrainWave DPU is a software programmable processor, programmed in single-threaded C, but we've added a number of instructions for serving DNNs, e.g., matrix multiply, convolution, nonlinear activations, embeddings. The processor is designed to use narrow precision format (float16) and easily flexible for extending to newer algorithms.

The microarchitecture of the processor, main portion is dedicated to matrix vector unit; matrix vector multiply, consisting of a number kernels on a tile of a larger matrix. Tiling gives us flexibility while maintaining performance. Other compute units are multifunction units; vector-vector operations, such as element-wise multiply, add and activation functions. Tying it all together is an on-chip network that lets us keep all the compute together at time.

Most of the chip is dedicated to matrix vector unit. It's composed of hundreds of multilane dot product units. Each of these dot product units is consists of tens of adds and muls. To keep them fed with data, each dot product unit is fed by a set of dedicated block rams.

Next, I'd like to show performance results for this architecture. Two years ago, we had a deployment of Stratix V FPGAs. It shows the effective teraflops of this format. 16 bit integer.. we've been playing with our own format Microsoft Floating Point. 4.5Tflops at MSFP5.8. These Stratix are pretty old.

(Demo for latest generation of FPGAs)

Looking at throughput oriented DPU, the latency is 65.81ms. With brainwave, latency is 0.98ms. Under 1 millisecond.

This was done on initial engineering silicon. For production silicon, we're expecting to get 12TOps at 16-bit integer. 90TOps for MSFP8. One question is how does numeric output affects output. Here is the normalized accuracy for three in-house text models, using GRU and LSTM. The orange bar shows what happens when you go to MSFP9, but we've developed a way to fine tune networks for this precision, and you see we recover our accuracy. We're working with MSFP8 and see similar results.

Project BrainWave is our project for accelerating DNNs at cloud scale. We hope it will be fast, friendly and cloud-scale, and expand capabilities of AI in the cloud, providing a way to run higher dimensional RNN networks for NLP and other great applications. We're planning to release to third parties, stay tuned.

Q: When you decrease batch size, what hardware are you evaluating? Hardware utilization as we decrease?

A: We stay highly utilized even as we decrease batch size; even at high batch size, we're still sending requests one by one. (Only one step will be processed?) Right.

Q: Regarding the FP9 and FP8, nine and eight being the number of bits used? (Yes) Is it in any way related to Flexpoint at Intel?

A: We developed this independently of flexpoint, and I'm not able to talk about our numeric format.

Q: In MS, do you really write Verilog for your FPGA, or do you use high level synthesis tool?

A: For this, we are writing System Verilog

Q: Batchnorm layers, which require batch computation; how do you put that onto the FPGA?

A: Part of the work of the compiler is to do splitting between CPU and FPGA. So things that are not amenable to FPGA, including batchnorm, we're still running them on CPU.

The below is a transcript of a talk by Virginia Smith on MOCHA, at the ML Systems Workshop at NIPS'17.

---

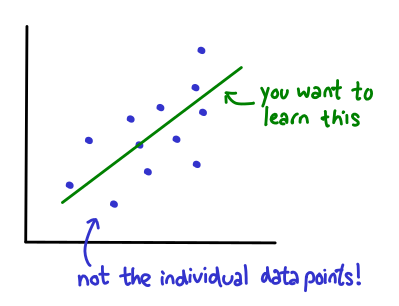
The motivation for this work comes from the way we think about solving ML problems in practice is changing. The typical ML workflow looks like this. You start iwth dataset and problem to solve. Say you want to build a classifier to identify high quality news articles. Next step is to select an ML model to solve the problem. Under the hood, to fit the model to your data, you have to select an optimization algorithm. The goal is to find an optimal model that minimizes some function over your data.

In practice, there's a very important part of the workflow that is missing. For new datasets, interesting and systems, the system and properties of system, play a large role in the optimization algorithm we select to fix. To give an example, in the past several years, data that is so large that must be distributed over multiple machines, in a datacenter environment. I've been thinking about how to perform fast distributed optimization in this setting, when data is so large.

But more and more frequently, data is not coming nicely packaged in datacenter. It's coming from mobile phones, devices, distributed across country and globe. Training ML in this setting is challenging. For one, whereas in datacenter you have hundreds to thousands, here you have millions and billions. Also, in datacenter, devices are similar capability; here, you have phones that are old, low battery, not connected to wifi. This can change ability to perform computation at any given iteration.

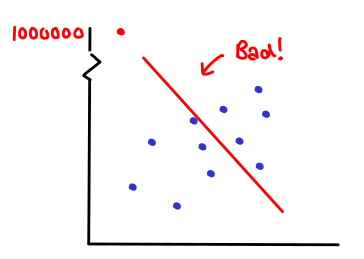
Additionally, there's heterogeneity in data itself. For privacy and computation reasons, data can become very unbalanced in network. And it can be non-IID, so much so that there can be interesting underlying structure to the data at hand. I'm excited because these challenges break down into both systems and statistical challenges. The one second summary of this work, thinking about both systems and statistical in this federated setting; the punchline is that systems setting plays a role not only in optimization algorithm but also the model we select to fit. IT plays a more important role in this overall workflow.

I'm going to go through how we holistically tackle systems and statistical challenges.

Starting with statistical. The goal is we have a bunch of devices generating data, could be unbalanced; some devices have more data than others. One approach used in past is fit a single model across all of this data. All of the data can be aggregated; you find one model that best achieves accuracy across all of the data simultaneously. The other extreme is you find a model for each of the data devices, and not share information. From systems point of view this is great, but statistically, you might have devices that are only ... that are poor in practice. What we're proposing is something between these two extremes. We want to find local models for each device, but share information in a structured way. This can be captured in a framework called multitask learning.

The goal is to fit a separate loss function for each device. These models can be aggregated in this matrix W, and the function of the regularizer, is to force some structure omega on it. This omega is a task relationship matrix, capturing interesting relationships, e.g., all the tasks are related and you want to learn weights, or most of the tasks are related and there are a few outliers, or there are clusters and groups, or there are more sophisticated relationships like asymmetric relationships. These can all be captured in multitask.

We developed a benchmarking set of real federated data. This includes trying to predict human activity from mobile phone, predict if eating or drinking, land mine, and vehicle sensor; distributed sensor to determine if a vehicle is passing by.

For these various datasets, we compared global, local and MTL. The goal is to fit a SVD model. For each data set, we looked at the average error across tasks, where each model is a task. What you can see is average error, for SVD, is significantly lower than global and local approaches. This makes sense because MTL is much more expressive; it lets you go between these extremes. What's interesting is that in these real data sets, it really helps. Reduction by half. This is a significant improvement in practice.

Given that we like to be using multitask learning to model data in federated environment, the next problem is figure out how to train this in distributed setting, thinking about massive distributed. In particular, the goal is to solve the following optimization objective. In looking how to solve this objective, we note that it's often common to solve for W and omega in an alternating fashion. When you solve for omega, it's centrally, you just need access to models. But W must be distributed because data is solved across devices. The key component how to solve this in practice is the W update. The challenge of doing this is communication is extremely expensive. And because of heterogeneity, you may have massive problems with stragglers and fault tolerance; e.g., someone who turns their phone off.

The high level idea for how we're doing this, take a communication efficient method that works well in data center, and modify it to work in federated setting. It will handle MTL as well as stragglers and fault tolerance.

What is the method we're using? The method we're using is COCOA, which is a state of the art method for empirical risk minimization problems. The thing that's nice about COCOa is it spans prior work of mini-batch and one-shot communication, by making communication a first class parameter of the method. Make it flexible as possible. It does it by not solving the primal formulation, but the dual. The dual is nice because we can easily approximate it by forming a quadratic approximation to the objective; and this more easily decomposes across machines.

To distribute this to federate setting, a key challenge is figuring out how to generalize it to the MTL framework. A second challenge; in COCOA, the subproblems are assumed to be solved to some accuracy theta. This is nice because theta varies from 0 to 1, where 0 is exact solve, and 1 is inexact. This can be thought of as how much time you do local communication versus communication. However, in fact, this is not as flexible as it should be in the federated setting. There is only one theta that is set for all iterations, a ll nodes. And because theta cannot be set exactly to one, it cannot handle fault tolerance, where there's no work performed at any iteration. Making this communication parameter much more flexible in practice.

JHow are we doing this? we developed MOCHA. The goal is to solve multitask learning framework; W and Omega in an alternating fashion. In particular, we're able to form the following dual formulation, similar to COCOA, so it decomposes. In comparison, we make this much more flexible assumption on subproblem parameter. This is important because of stragglers: statistical reasons, unbalance, different distributions, it can be very different in how difficult it is to solve subproblems. Additionally, there can be stragglers due to systems issues. And issues of fault tolerance. So this looks like a simple fix: we make this accuracy parameter more flexible: allow it to vary by node and iteration t, and let it be exactly 1. The hard thing is showing it converges to optimal solution.

Following this new assumption, and you can't have a device go down every single round, we show the following convergence guarantee. For L-Lipschitz loss, we get a convergence at 1/epsilon; for smooth models (logistic regression) we get a linear rate.

How does this perform in practice? The method is quite simple. The assumption is we have data stored at m different devices. We alternate between solving Omega, and W stored on each. While we're solving w update, it works by defining these local subproblems for machines, and calling solver that does approximate solution. This is flexible because it can vary by node and iteration.

In terms of comparing this to other methods, what we've seen is the following. Comparing MOCHA to CoCoA, compared to Mb-SDCA and Mb-SGD. We had simulation, with real data to see what would happen if we do it on wifi. We have simulated time and how close are to optimal. What you can see is that MoCHA is converging much more quickly to optimal solution, because MoCHA doesn't have the problem of statistical heterogeneity, and it's not bogged down by stragglers. This is true for all of the different types of networks; LET and 3G. The blue line and MOCHA and CoCOA, they work well in high communication settings, because they are more flexible. But compared to CoCOA, MOCHA is much more robust to statistical heterogeneity.

What's interesting is that if we impose some systems heterogeneity, some devices are slower than others, we looked at imposing low and high systems heterogeneity, MOCHA with this additional heterogeneity, it's a two orders of magnitude speedup to reach optimal solution.

And for MOCHA in particular, we looked at issue of fault tolerance. What we're showing here, we're increasing the probability a device will drop out at any distribution. Going up until there's half devices, we're still fairly robust to MOCHA converging, in almost the same amount of time. But what we see with green dotted line, of the same device drops out every iteration, it doesn't converge. This shows the assumption we made makes sense in practice.

The punchline is that in terms of thinking this new setting, training ML on these massive networks of devices, this is both a statistical and systems issue. We've addressed it in a holistic matter. Code at http://cs.berkeley.edu/~vsmith I also want to reiterate about SysML conference in February.

Q: When you compare global and local? Why is it always better than global?

A: The motivation why you want to use local model over global model, is that if you have a local data a lot, you might perform better. It boosts the overall sample size. I have some additional experiments where we took the original data, and skewed it even further than it already was. We took the local data, and there was less data locally, and they have global approaches. That's just a function of the data in the devices.

Q: I really like how your method has guarantees, but I'm wondering about an approach where you create a metalearning algorithm locally and have it work locally?

A: That's worth looking into empirically, since you can do fine tuning locally. What we were trying to do first was converge to exact optimal solution, but you might want to just work empirically well, would be good to compare to this setting.

The below is a transcript of a talk by Alex Beutel on machine learning database indexes, at the ML Systems Workshop at NIPS'17.

---

DB researchers think about there research differently. You have a system that needs to work for all cases. Where as in ML, we have a unique circumstance, I'll build a model that works well. In DB, you have to fit all.

To give an example of this is a B-tree. A B-tree works for range queries. We have records, key, we want to find all records for range of keys. 0-1000, you build tree on top of sorted array. To quickly look up starting point in range. What if all my data, all of the keys, from zero to million... it becomes clear, you don't need the whole tree above. You can use the key itself as an offset into the array. Your lookup is O(1), O(1) memory, no need for extra data structure.

Now, we can't go for each app, we can't make a custom implementation to make use of some pattern. DB scale to any application, we don't want to rebuild it any time.

But ML excels in this situation. It works well for a wide variety of distributions, learn and make use of them effectively.

This is the key insight we came to. Traditional data structures make no assumptions about your data. They work under any distribution, and generally scale O(n). Interestingly, learning, these data distributions, can offer a huge win. What we're trying to go to, is instead of scaling to size of data, we scale to complexity of it. With linear data, it's O(1). For other distributions, can we leverage this?

There are three dat structures underlying databases. There are B-Trees; range queries, similarity search. Main index. Hash maps for point lookups; individual records. This is more common throughout CS. And bloom filters, are really common for set-inclusion queries. Do I have a key. If your record is stored on disk, checking first if there's a record with that key is worthwhile. We're going to focus entirely on B-trees.

B-trees take a tree like structure with high branching factor. What makes it really effective is that it's cache efficient. You can store top level nodes in your cache where it's fast to look it up, maybe others in main memory, and the actual memory on disk. By caching the hierarchy appropriately, it makes it efficiently. At a high level, a B-tree maps a key to a page, some given place in memory. Once it finds that page, it will do some local search to find the particular range of that key. That could be a scan or binary search; we know the range will be the position from start of page to page size.

An abstract level, the Btree is just a model. It's taking the position of the key, and trying to estimate the position. What we have in this case, we want to search in this error range to find the ultimate record. At a high level, it would mean that we can't use any model. We need err_min and err_max. But we have all the data. If you have all the data, you know at index construction time, you know all the data you're executing against, and you can calculate what the model's min and max error is.

One interesting thing is this is just a regression problem. What you're really modeling is just the CDF. On the X axis on this plot here, the X axis is your keys, Ys your position. This is modeling where your probability mass is located; where your data is in the keyspace. CDFs are studied somewhat, but not a ton, in the literature. This is a nice new implication of research.

We thought, OK, let's try this out straightaway. Train a model, see how fast it is. We looked at 200M server logs, timestamp key, 2 layer NN, 32-width, relatively small by ML. We train to predict position, square error. A B-Tree executes in 300ns. Unfortunately, with the model, it takes 80000ns. By most ML model speeds, this is great. If you're looking at executing on server, great. But this doesn't work for a database.

There are a bunch of problems baked into this. TF is really designed for large models. Think about translation or superresolution images; these are hefty tasks. We need to make this fast for database level speed. Second, b-trees are great for overfitting. There's no risk of over-fitting in this context. They're also cache efficient; that's not looked at in ML. The last thing is local search in the end. Is that really the most effective way of ultimately finding that key? I'm skipping that part because it's fairly detailed, I'll focus on first three.

The first part is just the raw speed fo execution of ML model. This was built really by Tim, this Learning Index Framework program. What it does is it lets you create different indexes under different configurations. For one thing, it lets you do code compilation for TF, ideas from Tupleware, where you can take a linear model and execute it extremely quickly. We can also train simple models. Use TF for more complex gradient descent based learning; extract weights, and have inference graph be codegenned. And we can do a lot of autotuning, to find what the best model architecture is. We know ahead of time what the best training is. We can make pretty smart decisions about what works best.

The next problem is accuracy and sepeed. If I have 100M records, I narrow down quickly from 1.5M to 24K, with each step down this tree. Each one of those steps is 50-60 cycles to look through that page, and to find what the right branch is. So we have to get to an accurracy of 12000, within 500 mul/add, to beat these levels of hierarchy, which are in cache. This is a steep task. The question is what is the right model? a really wide network? Single hidden layer? This scales nicely, we can fit in 256 layer reasonably. We could go deeper... the challenge is we have width^2, which need to be parallelized somehow. The challenge is, how do we effectively scale this. We want to add capacity to the model, make it more and more accurate, with increased size, without becoming to.

We took a different approach, based on mixed experts. We'll have a key, have a really simple classifier. We get an estimate. Then we can use that estimate to find it at the next stage. Narrow down the CDF range, and try to be more accurate in the subset of space. It will still get key as input; given key, give position, but more narrow space of keys. We build this down, and we'll walk down this hierarchy. This decouples model size and complexity. We have a huge model, overfitting, but we don't have to execute all of the sparsity that you would have to do from a pure ML view. We can decouple it usefully. The nice thing we can do is fall back to B-trees for subsets that are difficult to learn in a model. The LIF framework lets us substitute it in easily. In the worst case, B-tree. Best case, more efficient.

The quick results version here, is we find we have four different data sets. Most are integer data sets; last one is string data set. We're trying to save memory and speed; we save memory hugely; these are really simple models. Linear with simple layer, with possibly two stages. We're able to get a significant speedup in these cases. Server logs one is interesting. It looks at a high level very linear, but there's actually daily patterns to this data accessed. Maps is more linear; it's longitudes of spaces. We created synthetic data that's log normal, and here we see we can model it effectively. Strings is an interesting challenge going forward; your data is larger and more complicated, building models that are efficient over a really long string is different; the overall patterns are harder to have intuition about. One thing really worth noting here, it's not using GPUs or TPUs; it's pureely CPU comparison. Apples-to-apples.

This is mostly going into the B-tree part. This is a regression model looking at CDF of data. We can use these exact same models for hash maps. With bloom filters, you can use binary classifiers. I have a bunch of results in the poster in the back.

A few minutes to talk about rooms for improvement. There are a bunch of directions that we're excited to explore. Obvious one is GPUs/TPUs. It's cPUs because that's when B-trees are most effective; but scaling is all about ML. Improving throughput and latency for models with GPUs, exciting going forward. Modeling themselves; there's no reason to believe hierarchy of models is the right or best choice; it's interesting to build model structures that match your hardware. Memory efficient, underlying architecture of GPUs. In the scale of ns we need for database. Multidimensional indexes; ML excels in high numbers of dimension; most things are not looking at a single integer feature. There's interesting question about how you map to multidimensional indexes that are difficult to scale. If we have a CDF, you can approximately sort it right there. And inserts and updates, assumed read-only databases. Large class of systems, but we get more data. How do we balance overfitting with accuracy; can we add some extra auxiliary data structures to balance this out?

Q: One thing is that when... this problem, we solved pretty well without ML. When we introduce ML, we should introduce new metrics. We shouldn't make our system more fragile, because distribution changes. What would be the worst case when distribution changes?

A: As the data becomes updated... in the case of inference and updates, there's a question about generalization. I think you could look at it from the ML point of view: statistically, test model today on tomorrows inserts. (It's a method. If I use this method, and then train it with data that I don't yet have... and do.) The typical extrapolation to future generalization of ML. Guarantees are hard. There will be a worst case that is awful... but the flip side, that's the ML side... generalization. There's also a point of view, I couple this with classic data structure. we coupled modeling with classic data structures: search, bloom filter case, so you don't actually have this work. You catch worst case.

Let me add to that. If you assume that the inserts follow the same distribution as trained model, then the inserts become all one operation. They're even better. Suppose they don't follow the same distribution? you can still do delta indexing. Most systems do do delta indexing. So inserts are not a big problem.

Q: (Robert) Most of the inputs were one or two real numbers, and outputs are a single real number. how does it work if you use a low degree polynomial, or a piecewise linear classifier on the different digits?

A: In the case of strings, it's not a single input. (Treat it as integer?) Well, it's possibly a thousand characters long. It's not the best representation. Different representations work really well. The last thing I want to say, piecewise linear could work, but when you run 10k, 100k submodels, it's slow. Hierarchy helps. Polynomials are interesting, depends on data source.

Q: Can you comment how bad your worst case is? Average numbers?

A: We specifically always have a spillover. The worst case is defaulting to typical database. We haven't had a case where you do worse, because we'll default to B-tree. (Deterministic execution?) Not inference time.

The below is a transcript of a talk by Ion Stoica on Ray, at the ML Systems Workshop at NIPS'17.

---

We've been working on it at Berkeley for more than one year. Over the past years, there's been tremendous progress in AI. Ad targeting, image&speech, many more. Many applications are based on supervised learning with DNNs. Supervised plus unsupervised are the two dominant approaches.

However, the next generation of AI applications will be very different. They're deployed in mission critical scenarios, need to continually learn from a rapidly changing env. Robotics, self driving cars, unmanned drones, dialogue systems. Implementing this new generation of AI applications requires a broader range of techniques. Stochastic optimization, parallel simulations, many more.

Ray provides a unified platform for implementing these approaches. To motivate Ray, I'll use reinforcement learning. RL learns by interacting with env. A policy mapping from state/observation to action that maximizes a certain reward. What are the reqs of RL? Many applications exhibit nested parallelism: search, where they use data parallel SGD, which then calls a component that does policy evaluation with a model to simulate, that runs in parallel on multiple CPUs. Second, these workloads can be highly heterogenous in hardware and time. Many of these computations require not only CPUs, but GPUs TPUs and FPGAs. Second, this computation can take wildly different times. Simulate a chess game: 3 moves to lose, or 50 moves to win or draw. And in robotics, we need to process in real time, processing the data from sensors in parallel, tens of ms.

Meeting these requirements is not easy. To meet these requirements, you need a system that is flexible and performant. Flexible: it should create and schedule tasks dynamically, and support arbitrary dependencies. Perf: it should scale to hundreds of nodes, sub-millisecond latency, millions of task, and efficiently share numeric data.

Next, I'm going to say how we achieve these challenges. Flexibility? We provide a very flexible model: dynamic tasks graphs. On top of this, we give the two models: parallel tasks and actors.

To talk about parallel tasks, here is Python code: one reads an array from a file, and the other adds two arrays. The code is simple: it creates two arrays a and b from file1 and file2, and sum them up. So now, parallelizing this program is quite easy. If we want to parallelize a function, in order to do that, we need to add a ray.remote decorator to each function. When we invoke these functions, you need to invoke remote method. Remove doesn't return object itself, just the object id. This is very similar to the futures abstraction. To get the actual object, you must invoke ray.get on the object id.

To get a better idea of how Ray is executing, let's execute a simple program. Assumes files stored on different nodes. When read_array on file1, it schedules read_array on the appropriate node. The remote call returns immediately, before the actual read finishes. This allows the driver to run the second task in parallel, running on the node on file 2, and launch the add remote function. All functions have been scheduled remotely, but none of them have finished. To actually get the result, you have to call ray.get on the result. This is a blocking call, you'll wait for the entire computation graph to be executed.

Tasks are very general, but they are not enough. Consider that you want to run a simulator, and this simulator is closed source. In this case, you do not have access to the state. You have state, action, simulations, to set up state in simulator, you cannot do it. So to get around this, there is another use case, where the state is too expensive to create. For example, DNNs on GPUs, in this case, you want to initialize it once, and reinitialize for each simulation.

In order to address these use cases, we add Actor abstraction. An actor is just a remote class. If you have a Counter, you mark it ray.remote, and the when you create the class or invoke methods, you use remote keyword. This is a computation graph for this very simple example. Notice the method invocations also return object identifiers. To get the results, you need to call ray.get on object identifiers. Ray also allows you to specify the number of resources, for actors and tasks.

To put things together, and provide a more realistic example, evaluation strategy, a scalable form of RL, by Salimans et al in OpenAI. In a nutshell, evolution strategy, tries lots of policies, and tries to see which runs best. This is highly parallel. So here is pseudocode for parallel strategies. A worker that does simulation and returns the reward, create twenty workers, and then 200, do 200 simulations, update policy. Again, if you want to parallelize this code, we have to add a bunch of remote, and now on the right hand side, you'll notice I'm also sharing the computation graph. When you invoke now, the Worker.remote, you create 20 remote workers to do it in parallel. And you invoke with the remote keyword. Again, notice that in this case, the results are not the rewards themselves, but they're ids to the reward objects. In order to get the rewards to get policy, you have to call ray.get.

This hopefully gives you a flavor how to program in Ray. Next time, I switch gears, presents system design of Ray; how Ray gets high performance and scalability.

Like many classic computing frameworks, it has a driver, and a bunch of workers. Driver runs a program, worker runs task remotely. You can run and write a bunch of actors. The drivers actors on the same node, they share the data, on shared memory, and the workers and actors of cross nodes, share through distributed object store we built. Each node has a local scheduler, so when a driver wants to run another task, the local scheduler tries to schedule it locally. If it cannot schedule it locally, it invokes global scheduler, and it will schedule another node that has resources. Actor, remote method. Finally, what we do, and one essential part of the design, is we have a Global Control State. It takes all of the state of the system, and centralizes it. The metadata for the objects, in objects table, function. This allows system to be stateless. All these other components can fail, you can bring them up, get the most recent data from global control state. It also allows us to parallelize the global scheduler, because these replicas are going to share the same state in the GCS.

Another nice effect of having a GCS is that it makes it easy to build a bunch of profiling and debugging tools.

This design is highly scalable. Let me try to convince you why this is. To make GcS scalable, we just shard it. All these keys are pseudorandom, so it's easy to shard and load balance. The scheduler as you see is distributed; each node has a local scheduler, and Ray tries to schedule tasks which are spawned by a worker/driver on another task that is locally. The global scheduler, becomes a bottleneck, we can also replicate it. Finally, in systems, even if scheduler is super scalable, in Spark, there's another bottleneck: only the driver can launch new tasks. In order to get around that, we allow in Ray the workers and actors to launch tasks. Really, there is no single bottleneck point.

A few words about implementation. The GCS is implemented with Redis. For object store, we leverage Apache Arrow. For fault tolerance, we use lineage based fault tolerance like Spark. Actors are part of task graph; methods are treated as tasks, so we have a uniform model for providing fault tolerance.

So now some evaluation results. This plot represents the number of tasks per second, and you can see the number of nodes; it scales linearly. You can schedule over 1.8 M/s. Latency of local task execution is 300us, the latency of remote task is 1ms. This plot illustrates fault tolerance. You may ask why you care about fault tolerance? The problem is you need in your program that the simulation may not finish; this makes the program far more complicated, even if you're willing to ignore some results. Here, on this axis, you have the time in seconds, you have two y axes, number of nodes in system, and the throughput. As you can see, the number of nodes is starting at 50, then 25, then to 10, and goes back to 50. In the red area, you show the number of tasks per second; it follows as you may expect, the number of nodes in the system. If you look a little bit, there are some drops; every time, you have a drop in the number of tasks. It turns out this is because of the object reconstruction. When some nodes go away, you lose the objects on the node, so you have to reconstruct them. Ray and Spark reconstruct them transparently. With blue, you can see the re-executed tasks. If you add them, you get a very nice filling curve.

Finally, for evolution strategies, we compared with reference ES from... we followed the OpenAI, and on the X axis, you have number of CPUs, mean time to solve the particular problem; simulator, learning to run, there are three points to notice. One is, as expected, as you add more CPUs, the time to solve goes down. The second is that Ray is actually better than the reference ES, better results, even though the reference ES is specialized for beating. Third, for a very large number of CPUs, ref couldn't do it, but Ray could do better and better. I should add that Ray takes half the amount of code, and was implemented in a couple of hours.

Related work: look, in this area, there are a huge number of systems, that's why you are here, lots of systems. Ray is complimentary to TF, MXNet, PyTorch, etc. We use these systems to implement DNNs. We integrate with TF and PyT. There are more general systems, like MPI and Spark; these have limited support for nested parallelism; computation model, and they have much coarser grained tasks.

To conclude, Ray is a system for high performance and flexibility and scalability. We have two libraries on top of Ray: RLlib and Ray Tune. It's open source, please try, we'd love your feedback. Robert, Philip, Alex, Stephanie, Richard, Eric, Heng, William, and many thanks to my colleague Michael Jordan.

Q: In your system, you also use actor; actor is built up on shared memory. Do you have separate mailbox for actors? How do you do that?

A: No, the actors communicate by passing the argument to the shared object store.

Q: What is the granularity of parallelism? Is it task atomic, or do you split task?

A: The task granularity is given by what is the overhead for launching a task and scheduling the task. The task you see, we are targeting task, low and few ms. The task is not implementing something like activation function. we leave that job to much better frameworks. And a task is executing atomically, a method, in the actors, are serialized.

Q: Question about fault tolerance: in Spark, when you don't have a response for some time, it says this node died. Here, the task is much more, because NN, something like that. So we don't have the same time.

A: We do not do speculation; implicit speculation in Ray, for the reason you mentioned.

Q: Can you give me more details on the reference implementation, doesn't scale

A: The reference implementation, it's the OpenAI implementation, Robert here can provide you a lot more detailed answers to that question.